» Contribute     » Cast     » Archive     » Authors     » Search     » Random    » Resources     » Statistics     » Forum     » RSS Feed     Updates Mon, Wed, Fri when available

No. 82: Love Between Parallel Lines On A Cartesian Plane

First | Previous | 2009-03-30 | Next | Latest

First | Previous | 2009-03-30 | Next | Latest

Permanent URL: https://mezzacotta.net/owls/?comic=82

Strip by: Matt Albrecht

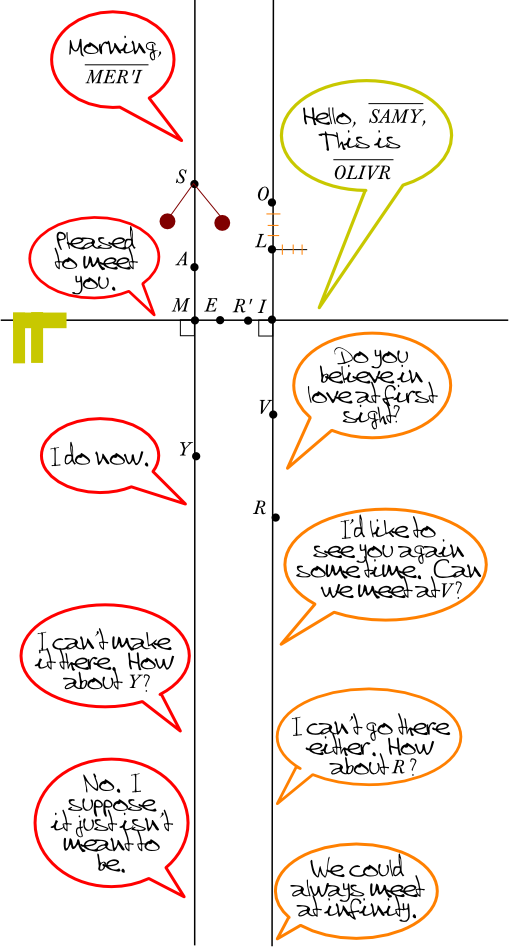
Setting: A cartesian plane. Line MER'I intersects lines SAMY and OLIVR at points M and R, respectively, forming a right angle at each intersection.

SAMY: Morning, MER'I.
MER'I: Hello, SAMY. This is OLIVR.
SAMY: Pleased to meet you.
OLIVR: Do you believe in love at first sight?
SAMY: I do now.
OLIVR: I'd like to see you again. Can we meet at V?
SAMY: I can't make it there. How about Y?
OLIVR: I can't go there either. How about R?
SAMY: No. I suppose it just isn't meant to be.
OLIVR: We could always meet at infinity.

The author writes:

I chose a Cartesian plane for a reason. There's an expression which I recall hearing in grade school, stating that "parallel lines meet at infinity." Unfortunately, this isn't true for Euclidean geometry. Most simply put, the parallel postulate of Euclid states that parallel lines don't meet.

This gives our two linear lovers a bittersweet parting.

In everyday terms, most people associate infinity with metaphysical concepts such as deities. In mathematics, there are actually several meanings for infinity (symbolised by ∞), depending on its context. Most people are familiar with the context of number systems. My favourite definition of this reads:

If x is a number, then x < ∞.

which means that infinity isn't a number, but rather a concept to describe the upper limit of a number. If we look at our parallel lines from an analytical geometry perspective, where a line is defined by a function, such as:

y = 12 x + 1

it's quite clear why two lines can't meet at infinity - the function only deals with numbers, and not infinity.

Another form of infinity comes from when there's a pattern of two things moving towards each other. For example, if you divide one by bigger and bigger numbers, you find it getting really close to zero, but it never reaches zero. In this situation, mathematicians say that this converges to zero at infinity.

The last context deals with sizes of things. We can say that the number of counting numbers (1, 2, 3, ...) is infinite. We can keep on counting and never reach the end.

However, let's look at that example above of dividing 1 by bigger and bigger numbers. This gives you numbers that get really, really close to zero, with a bunch of zeros behind the decimal point, but never quite make it to zero. For these numbers (called "real numbers"), we can't even count the numbers between zero and one. Heck, we can't even find the first number after zero to start our counting!

It turns out that these are countably infinite and uncountably infinite, respectively, in size. These are formally described by the Aleph numbers, which show, formally, yet another meaning of infinity, where now there are different infinities.

Our parallel lines have a long road ahead of them, but I hope they give up on meeting each other and try to intersect a different line.