Hi all. Sorry for the long hiatus in writing new articles. I haven’t forgotten or abandoned this project! I still have my list of over 100 proofs to work through. I’ve just been busy with other activities that haven’t given me enough time to sit and write new articles. I do plan to get back to it in the not-too-distant future, but it’s hard to say exactly when.
Thanks for your patience in the meantime!
There is a fairly straightforward experiment that we could do to establish that the Earth is a globe: Set off an enormous bomb.
Something of explosive power equivalent to 5-10 megatons of TNT. This is around 500 times as powerful as the Hiroshima atomic bomb. Ivy Mike, the first first full-scale thermonuclear hydrogen bomb detonation by the United States would serve nicely, with its yield of just over 10 megatons. The explosion of such a bomb would create a huge shockwave in the atmosphere, which would propagate outwards at the speed of sound in all directions.
This explosive shockwave would create a momentary barometric pressure spike as it passed by weather stations. Nearby stations would see a large pressure spike, while stations thousands of kilometres away would see a spike of the order of a millibar or two. This is strong enough that barometers all over the world should be able to detect the pressure wave passing through the atmosphere. You could then map the arrival time of the pressure wave, emanating in concentric circles from the bomb’s detonation point.
At the point on the Earth antipodal to where the bomb detonated, exactly on the opposite side of the globe, the pressure wave would converge from all directions approximately 16 hours later. This would demonstrate that there is an antipodes, a point on the opposite side of the spherical Earth. This phenomenon of the converging shockwave would not be observed on a flat Earth.
Unfortunately, this experiment is ethically dubious at best.
On 14 January, 2022, the volcano Hunga Tonga erupted explosively, approximately 65 km north of Tonga’s main island of Tongatapu. As I write this a week later, the scale of the destruction and damage to Tonga is still being assessed. According to various estimates, the explosion released somewhere in the range of 5-10 megatons of energy, making it the largest volcanic explosion of the 21st century to date. The eruption and the resulting tsunamis are of course a human tragedy, but they also provide opportunities for knowledge and understanding.
The explosion occurred at 17:15 local time on 14 January (04:15 UTC, 15 January). Soon after, meteorological stations across the world were recording changes in barometric pressure due to the shockwave. The Australian Bureau of Meteorology released this trace of mean sea level pressure changes caused by the eruption.
The figure shows the propagation time delays as the shockwave travels at the speed of sound. Norfolk Island is 1900 km from Tonga and the wave arrived after about 1 hour 50 minutes. This gives the average speed of sound to be around 330 m/s, which is within the expected range. Perth is 6840 km from Tonga and the wave arrived after a little more than 6 hours. This gives the average speed of sound to be around 310 m/s, which is also within the expected variation given pressure and humidity differences.
Australia is relatively close to Tonga, globally speaking. The UK Met Office also released traces of barometric pressure as recorded at a couple of weather stations near Oxford in the UK.
The wave arrived after about 14.5 hours. Given the Tonga-Oxford distance of 16,600 km, this gives a speed of 314 m/s, again well within the expected range. More interesting is the arrival of a second wave a little less than 7 hours later. If we use the same speed of sound, this gives a propagation distance of 24,300 km. This is more than half way around the world. In fact, within the expected range of variation of propagation speed, this is close enough to the distance from Tonga to Oxford, travelling in the opposite direction—the long way around the world.
And this is exactly what we are seeing here. The first recorded wave travelled the shortest path from Tonga to Oxford, while the second wave went in the opposite direction, the long way, and so took 7 hours longer to arrive.
This by itself is strong evidence of the spherical shape of the Earth. But even more dramatic are videos showing propagation of the atmospheric shock wave, as imaged by weather satellites. In the days after the eruption, several meteorologists and atmospheric scientists downloaded publicly available images from geostationary weather satellites. They took infrared images, which show cloud patterns even in night time, and subtracted pairs of images taken at intervals 15 minutes apart, to show the differences between the state of the atmosphere in that time frame.
One resulting video using a satellite over the Pacific Ocean shows the site of the explosion in Tonga and the shockwave rippling outwards in concentric circles on the globe. As the shockwave propagates, you can see it getting weaker.
A second video uses a satellite over Africa. This shows the shockwave converging on the opposite side of the world. The eruption occurred at latitude 20.550°S, longitude 175.385°W. The antipodal point on the opposite side of the world is thus latitude 20.550°N, longitude 4.615°E, in southern Algeria. And in this video, you can see the shockwave converging on that point in Algeria, and then expanding again, producing the second wave that was later recorded in Oxford. (This video is sped up relative to the first video, to make it easier to see the shockwave propagation, because it is significantly weaker and more difficult to see at the slower speed.)
This provides clear visual evidence that the shockwave expands from one point on the Earth’s surface and, after propagating in all directions at approximately the same speed, it converged back to a point on the opposite side of the globe, equidistant from the origin in all directions.
We may not be able to reproduce the experiment with a bomb in good conscience, but nature often provides fascinating ways for us to test our understanding of the world and the universe.
With Proof 45 I asked readers if they would help perform an experiment to measure the parallax of the moon, and so derive a figure for the radius of the Earth. The experiment was more demanding than when we used Eratosthenes’ method, requiring helpers to stay up for much of the night and take specific photos of the moon and stars. Unfortunately this meant that only two readers contacted me to help perform the experiment, which simply isn’t enough to do it successfully, given the vagaries of weather. (The planned day was rainy here so I couldn’t be a third observer.)
So we don’t have any results to report. I may try this experiment again some time in the future, but I’ll need a significantly higher response from readers if we’re to get it to work.
If you hold your arm outstretched before you with a finger raised in front of some object in the distance, and you close one eye and align your finger so that it looks directly in front of a particular object, then close that eye and open the other eye, then your finger will appear to have moved sideways. This is because your left eye and your right eye are not in the same position, so lining up your finger with an object using one eye means that it is not aligned with the other eye. This effect is known as parallax.
Parallax is a purely geometric effect, caused by the divergence of straight lines drawn from a common object to two different viewpoints. We make use of parallax unconsciously, our brains using the different visual information from our two eyes to give us the sensation of viewing a three dimensional world around us.
We’ve discusses parallax briefly in Proof 28: Stereo imaging. Here’s a repeat of the diagram illustrating parallax (with a dog instead of a finger).
Illustration of parallax. The dog is closer than the background scene. Sightlines from your left and right eyes passing through the dog project to different areas of the background. So the view seen by your left and right eyes show the dog in different positions relative to the background. (The effect is exaggerated here for clarity.)
Parallax can be observed wherever you look at two objects at different distances. If you measure the distance between the two viewpoints and the angle between the two different sightlines, and you know the distance to one of the objects, then by simple geometry you can calculate the distance to the other object. A handy approximation is that if one object is much further away than the near object, then you can assume it is infinitely far away, which simplifies the geometry and produces only a negligibly small error.
Parallax can be used in this way to measure the distance to some astronomical objects, namely those objects close enough to Earth to have a measurable parallax angle when compared to the positions of distant stars. This includes objects in our solar system, as well as the very nearest stars. The European Space Agency satellite Hipparcos measured parallaxes of around 100,000 stars, using observations made six months apart so that the two different viewpoints are on opposite sides of the Earth’s orbit, a viewpoint separation baseline of 300 million km. Even with this enormous baseline, the parallax angle of stars is less than one second of arc, requiring precise instruments to even detect. However, there is a much closer celestial object for which the parallax is readily visible with a smaller baseline: the moon.
The moon is not close enough to see parallax from your left and right eyes. It is however close enough that photos of the moon taken from different locations on the Earth at the same time show the moon significantly shifted against the background of stars. With viewpoints on opposite sides of the Earth, the parallax angle of the moon is about one degree – it varies slightly due to the moon’s elliptical orbit.
Illustration of parallax of the moon, as seen from four different points on the Earth’s surface at the same time. Viewed from the North Pole, the moon occults stars in the constellation of Pleiades, but viewed from other locations the moon does not occult the same stars. (Public domain image from Wikimedia Commons.)
Using such observations of the moon, and knowing how far apart the viewpoints are, you can calculate the distance to the moon with high accuracy. But you can also do the reverse calculation: if you know the distance to the moon, you can calculate how far apart the viewpoint locations are. As mentioned in Proof 32: Satellite laser ranging, we have an independent method of measuring the distance to the moon, to an accuracy of better than a millimetre, by bouncing lasers off reflectors left on the moon by the Apollo astronauts. Before 1969, we could also measure the distance to the moon using radar ranging, to an accuracy of about 1 km.[1][2]
Incidentally, the 1965 paper by Yaplee, et al.[2], also includes a calculation of the radius of the Earth from the radar ranging data, as it comes out as a variable that can be solved for if you know the acceleration due to gravity at Earth’s surface and the ratio of the Earth’s mass to the moon’s mass, which were known at the time. Given their radar results, the authors calculate the Earth’s equatorial radius to be 6378.167 km, only 30 metres different to the current reference value of 6378.137 km. (Obviously a result unobtainable if you assume the Earth is flat.)
Diagram from Yaplee, et al., showing the geometry of the Earth-moon system for the purpose of determining the distance to the moon using radar ranging. (Figure reproduced from [2].)
So even without using parallax we know the distance to the moon. This means we can do the reverse calculation, and figure out how far apart different viewpoints (such as the North Pole and South Pole) are. Any two antipodal points, on opposite sides of the Earth, are about 20,000 km apart – as the crow flies, or as a traveller would have to cover to go from one point to the other. However if you calculate how far apart those points are using lunar parallax, you find that they are actually about 12,730 km apart. Why such a big discrepancy?
The simplest explanation for this is that the Earth’s surface is curved, with 20,000 km representing the semi-circumference and 12,730 km being the diameter (twice the radius) of curvature. If the Earth were flat, then the calculated distance between two points for which the travel distance is 20,000 km would also have to be 20,000 km.
Experiment:
With your help, we can perform a lunar parallax experiment to see if the Earth is flat or not, and to measure its radius if we assume it’s spherical. All we need to do is take some photos of the moon and the background stars at the same time, from different places around the world. The best time to do this is when the moon is up most of the night, which means a full moon. The next full moon after this is published occurs on 31 October 2020 at 14:49 UTC. This means the night of 31 October-1 November is perhaps the best time to do this. However I will be travelling that night and unable to participate myself, and perhaps many people will be busy with Halloween activities. So I propose we do it on the following night: the evening of 1 November into the morning of 2 November. This has the slight advantage that the moon rises and sets a bit later, meaning it will be easier for people to get a photo just before sunrise, which is vitally important as I shall explain.
I could set one specific time for everyone to take photos, but that would not be ideal, because half the planet won’t be able to see the moon at the given time, and there will also be places where the moon is up, but it will be too light to see stars. So instead, we will take photos on the hour, every hour, for as much of the night as you can manage. For this to provide useful data, the photos must satisfy the following conditions:
1. We absolutely must have some photos taken in the midnight-to-sunrise period, and preferably some taken just before sunrise (e.g. 4 or 5 a.m.). This is so they can be matched with photos taken in the evening in different time zones. If everyone just takes a few photos in the evening and goes to bed, this won’t work. It’s better if you take a shot before going to bed, and then drag yourself out of bed for another shot before sunrise. I realise not everybody will want to do this, but at least some of you will need to. If you really can’t manage it, please stay up as late as possible and shoot every hour until you do go to bed.
2. Stars must be visible in the photos. Enough stars that we can recognise the constellations, not just 3 or 4 stars. This means you will need to overexpose the moon quite severely. That’s fine, we’re not interested in seeing details of the moon, as long as we can figure out where the middle of the moon is. This means you shouldn’t zoom right into the moon – a moderate focal length lens will work fine, around 50-100 mm for a 35 mm camera. Go out some night beforehand and practice taking a photo of stars to figure out the correct exposure.
Some example photos that would be fine for this experiment:
If you’re interested in taking photos for this experiment, please contact me (dmm [at] dangermouse.net) to register your interest, with your location and a list of hours that you would be willing to be awake to take photos. Maybe send me a sample photo you’ve taken showing stars. I’ll coordinate the list and make sure we have enough people to make the experiment worthwhile, and will let everyone know a few days beforehand whether we will go ahead, or if we should postpone the date until we get more people. And if we do go ahead, what hours will be most valuable for you to take photos.
References:
[1] Yaplee, B. S., Bruton, R. H., Craig, K. J., Roman., N. G. “Radar echoes from the moon at a wavelength of 10 cm.” Proceedings of the Institute of Radio Engineers, 46(1), p. 293-297, 1958. https://doi.org/10.1109/JRPROC.1958.286790
[2] Yaplee, B. S., Knowles, S. H., Shapiro, A., Craig, K. J., Brouwer, D. “The mean distance to the Moon as determined by radar.” In Symposium-International Astronomical Union, 21, p. 81-93, Cambridge University Press, 1965. https://doi.org/10.1017/S0074180900104826
I’ve still been a bit too busy and distracted to write more proofs recently. However, I mentioned this project to my mother, and she expressed a keen interest in learning more. Unfortunately her eyesight has deteriorated and her lifelong avid reading habit has necessarily been converted to audiobooks.
So I decided to record the proofs to audio files and send them to her. She is thoroughly enjoying them! And now I’m sharing the audio files here on the site. I’ve recorded an Introduction, and the first six Proofs so far – you can access the audio files from the main Index page.
Some of them are verbatim readings of the article. Others that rely strongly on diagrams I have edited slightly so that they flow without requiring reference to the diagrams. I hope that some of you find these files useful.
And I do expect to have a bit more free time to be able to start writing some more proofs in the next week or two.
We’ve discussed continental drift and plate tectonics in Proof 22. Plate tectonics. There’s another aspect of plate tectonics that was mentioned in passing there, which deserves some further attention. Proof 22 stated:
But as technology advanced, detailed measurements of the sea floor were made beginning in the late 1940s, including the structures, rock types, and importantly the magnetic properties of the rocks.
That last one, the “magnetic properties”, was the piece of evidence that really cemented continental drift as a real thing.
We pick up the history in 1947, when research expeditions led by American oceanographer Maurice Ewing established the existence of a long ridge running roughly north-south down the middle of the Atlantic Ocean. They also found that the crust beneath the ocean was thinner than that beneath the continents, and that the rocks (below the seafloor sediment) were basalts, rather than the granites predominantly found on continents. There was something peculiar about the Earth’s crust around these mid-ocean ridges. And over the next few years, more ridges were found in other oceans, revealing a network of the structures around the globe. The system of mid-ocean ridges had been discovered, but nobody yet had an explanation for it.
Meanwhile, from 1957, the Russian-American oceanographer Victor Vacquier took World War II surplus aerial magnetometers that had been used to detect submerged submarines from reconnaissance aircraft, and adapted them for use in submarines to examine the magnetic properties of the sea floor. It was well known that basalt contained the mineral magnetite, which is rich in iron and can be strongly magnetised.
What Vacquier found was unexpected and astonishing. In a survey of the Mendocino Fault area off the coast of San Francisco, Vacquier discovered that the sea floor basalt was not uniformly magnetised, but rather showed a distinctive and striking pattern. The magnetism appeared to be relatively constant along north-south lines, but to vary rapidly along the east-west direction, causing “stripes” of magnetism running north-south.[1]
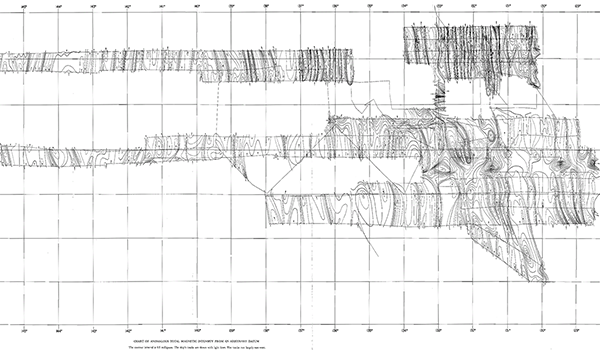
Map of magnetic field measurements on the sea floor near Mendocino Fault, showing strong north-south striping of the magnetic field. (Figure reproduced from [1].)
Follow up observations showed that the stripes were not localised, but extended over large regions of the ocean.[2][3].
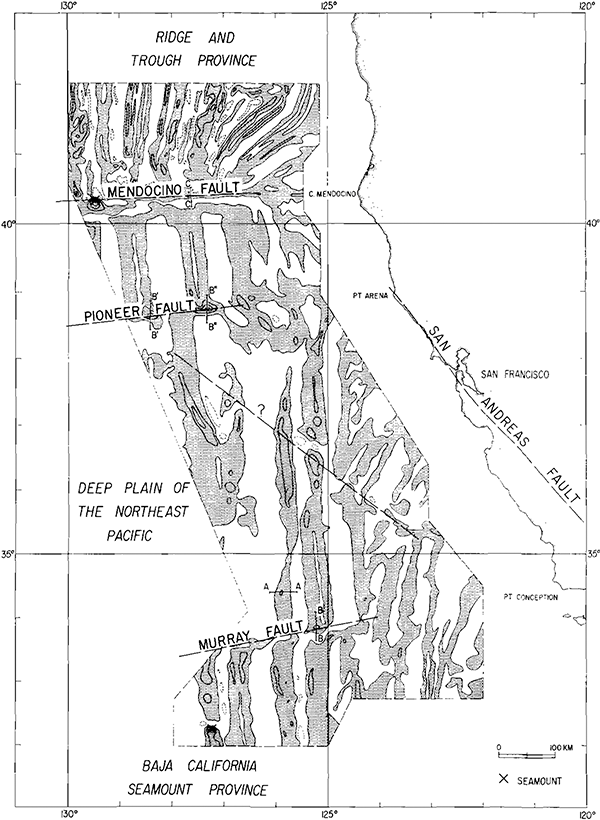
Map of magnetic anomalies on the sea floor off California. Shaded areas are positive magnetic anomaly, unshaded areas are negative. (Figure reproduced from [2].)
In fact, these magnetic “zebra stripes” were present pretty much everywhere on the floor of every ocean. They weren’t always aligned north-south though – it turned out that they were aligned parallel to the mid-ocean ridges. The early discoverers of this odd phenomenon had no explanation for it.
Returning to the mid-ocean ridges, American oceanographer Bruce Heezen wrote a popular article in Scientific American in 1960 that informed readers of the recent discoveries of these enormous submarine geological features.[4] In the article, he speculated that perhaps the ridges were regions of upwelling material from deep within the Earth, and the sea floors were expanding outwards from the ridges. Heezen was not aware of any mechanism for regions of Earth’s crust to disappear, so he suggested that the Earth might slowly be expanding, through the creation of new crust at the mid-ocean ridges.
Although Heezen’s idea of an expanding Earth didn’t take hold, his idea of upwelling and expansion along the mid-ocean ridges was quickly combined with existing proposals (that Heezen had overlooked) that crust could be disappearing along the lines of deep ocean trenches, as parts of the Earth moved together and were subducted downwards. The American geologists Harry Hammond Hess and Robert S. Dietz independently synthesised the ideas into a coherent theory of continental drift, combining the hypotheses of seafloor spreading and ocean trench subduction to conclude that the Earth was not changing size, but rather it was fractured into crustal plates that slowly moved, spreading apart in some places, and colliding and subducting in others.[5][6]
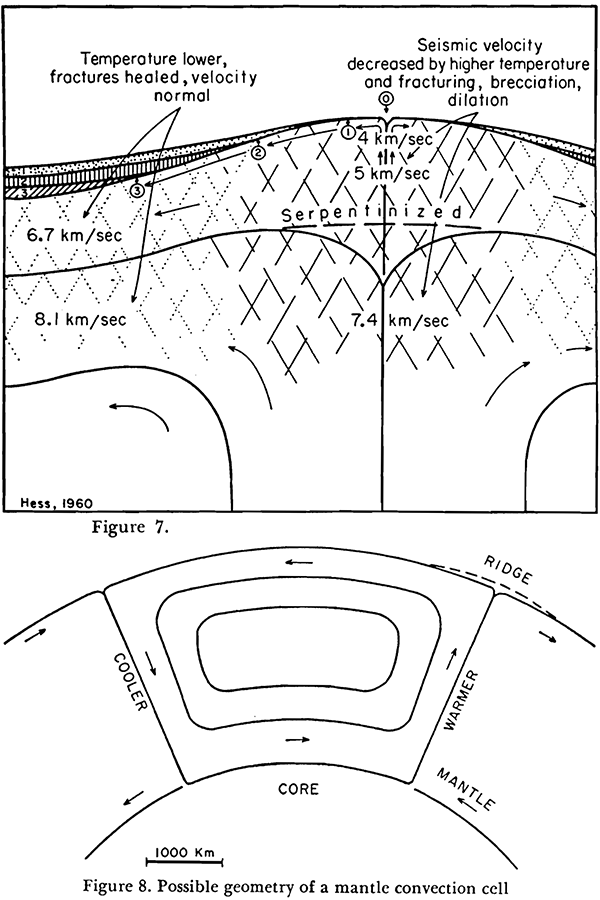
Earliest diagrams of proposed mantle convection cells causing continental drift, with upwelling at mid-ocean ridges causing seafloor spreading, by Harry Hammond Hess. Figure 7 (top) shows the detailed structure of a mid-ocean ridge, with measured seismic velocities (the speed of seismic waves in the rock) in various regions. Hess proposed that the observed lower speeds in the central and upper zones were caused by fracturing of the rock as it deforms during the upwelling, plus higher residual temperature of the upwelled material. Figure 8 (bottom) shows Hess’s proposed mantle convection cells. (Figures reproduced from [6].)
So by the 1960s, most of the observational pieces of this puzzle were in place. However, the unifying theory that would explain it all still required some synthesis, and acceptance of some unestablished hypotheses. This synthesis was again put together independently by two different groups of geologists: the Canadian Lawrence Morley, and the English Ph.D. student Frederick Vine and his supervisor Drummond Matthews. Morley wrote two papers and submitted them to Nature and the Journal of Geophysical Research in 1963, but both journals rejected his work as too speculative. Vine and Matthews thus received publication priority when Nature accepted their paper later in 1963.[7]
The geologists pointed out that if new rock was being created at the mid-ocean ridges and then spreading outwards, then the seafloor rocks should get progressively older the further away they are from the ridges. Each one of the magnetic zebra stripes running parallel to the ridges then corresponds to rocks of the same age. If, they conjectured, the rocks record the direction of the Earth’s magnetic field when they were formed, and for some reason the Earth’s magnetic field reversed direction periodically, that would explain the existence of the magnetic stripes.
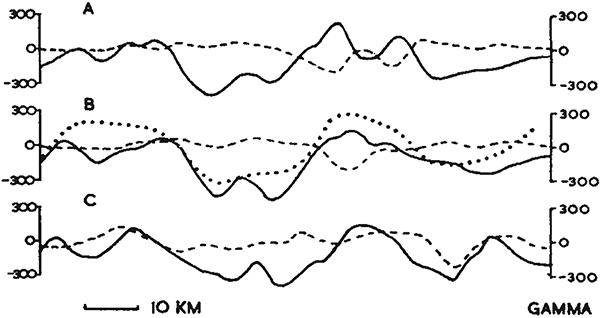
Diagram by Vine and Matthews showing the observed magnetic field strength of the sea floor rocks measured across the Carlsberg Ridge in the Indian Ocean, showing positive and negative regions (solid lines), computed magnetic field strength under conventional (for the time) assumptions (dashed lines), and computed field strength assuming 20 km wide bands in which the Earth’s magnetic field has been reversed. The periodic field reversal matches the observed magnetism much better. (Figure reproduced from [7].)
As with Morley’s rejected papers, this paper was treated with scepticism initially, because it relied on two unproven conjectures: (1) that the rocks maintain magnetism aligned with the Earth;’s magnetic field at the time of solidification and, much more unbelievably, (2) that the Earth’s magnetic field direction reverses periodically. For some geologists, this was too speculative to be believe.
One test of Vine and Matthews’ seafloor spreading hypothesis would be to measure the age of the sea floor using some independent method. If the rocks were found to get progressively older the further away they are from the mid-ocean ridges, then that would be strong evidence in favour of the theory. As it happens, it’s possible to date the age of rocks formed from magma, using a method of radiometric dating known as potassium-argon (K-Ar) dating. Potassium is a fairly common element in rocks, and the isotope potassium-40 is radioactive, with a half-life of 1.248×109 years. Most of the potassium-40 decays to calcium-40 via beta decay (see Proof 29. Neutrino beams for a recap on beta decay), but just over 10% of it decays via electron capture to the inert gas argon-40. Argon is not present in newly solidified rock, but the argon produced by decaying potassium-40 is trapped within the crystal structure. Since the decay rate is known very precisely, we can use the measured ratio of potassium to argon in the rock to determine how long it has been since it formed, for timescales from several million to billions of years.
In the mid-1960s, oceanographers and geologists began drilling cores and taking basalt samples from the sea floor and measuring their ages.[8] And what they found matched the prediction from seafloor spreading: the youngest rocks were at the ridges and became progressively older towards the edges of the oceans.
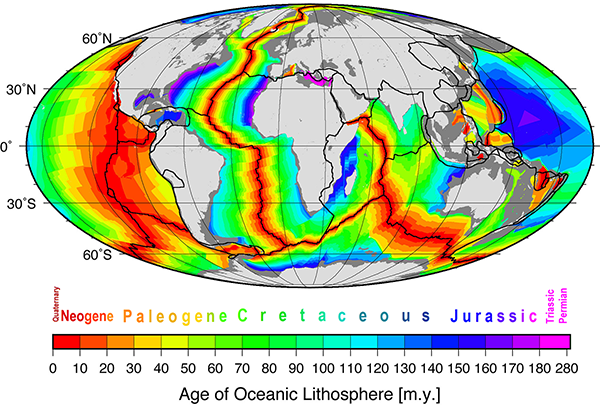
Diagram of the age of oceanic crust. The youngest rocks are red, and found along the mid-ocean ridges. Rocks are progressively older further away from the ridges. (Figure reproduced from [9].)
This was exactly what Vine and Matthews predicted. Belatedly, Morley also received his due credit for coming up with the same idea, and their proposal is now known as the Vine-Matthews-Morley hypothesis. The magnetic striping of the ocean floors is caused by the combination of the spreading of the ocean floors from the mid-ocean ridges, and the periodic reversal of Earth’s magnetic field.
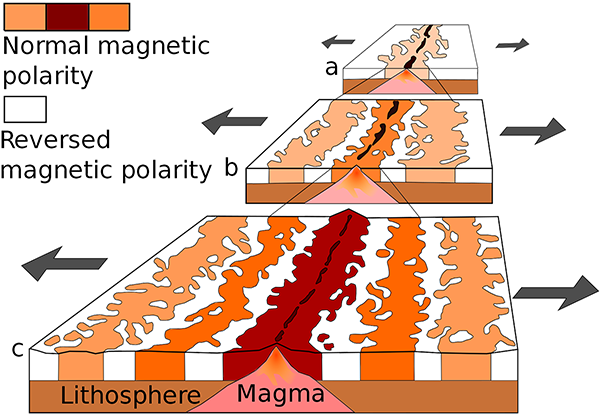
Generation of magnetic striping on the sea floor. As the sea floor spreads, and the Earth’s magnetic field reverses from time to time, stripes of different magnetic polarity are created and spread outwards. (Public domain image by the United States Geological Survey, from Wikimedia Commons.)
Odd reversals of magnetic fields in continental rocks had been noticed since 1906, when the French geologist Bernard Brunhes found that some volcanic rocks were magnetised in the opposite direction to the Earth’s magnetic field. In the 1920s, the Japanese geophysicist Motonori Matuyama noticed that all of the reversed rocks found by Brunhes and others since were older than the early Pleistocene epoch, around 750,000 years ago. He suggested that the Earth’s field may have changed direction around that time, but his proposal was largely ignored.
With the impetus provided by the seafloor spreading idea, geologists began measuring magnetic fields and ages of more rocks, and found that they matched up with the ages of the field reversals implied by the sea floor measurements. Progress was rapid and the geological community turned around and developed and adopted the whole theory of plate tectonics within just a few years. By the end of the 1960s, what had been ridiculed less than a decade earlier was mainstream, brought to that status by the confluence of multiple lines of observational evidence.
It had been established that the Earth’s magnetic field must reverse direction with periods of a few tens of thousands to millions of years. The remaining question was how?
Up until the development of plate tectonics, the origin of the Earth’s magnetic field had been a mystery. Albert Einstein even weighed in, suggesting that it might be caused by an imbalance in electrical charge between electrons and protons. But plate tectonics not only raised the question – it also suggested the answer.
The core of the Earth was known to be mostly metallic (see Proof 43. The Schiehallion experiment). If there are convection currents in the mantle, then heat differentials at the boundary should also cause convection within the core. The convecting metal induces electrical currents, which in turn produce a magnetic field. In short, the core of the Earth is an electrical dynamo. And because the outer core is liquid, the currents are unstable. Modern computer simulations of convection in the Earth’s core readily produce instabilities that act to flip the polarity of the magnetic field at irregular intervals – exactly as observed in the record of magnetic striped sea floor rocks.
Computer simulations of convection currents in the Earth’s core and resulting magnetic field lines. Blue indicates north magnetic polarity, yellow south. The left image indicates Earth in a stable state, with a magnetic north pole at the top and a south at the bottom. The middle image is during an instability, with north and south intermingled and chaotic. The right image is after the unstable period, with the north and south poles now flipped. (Public domain images by NASA, from Wikimedia Commons.)
So we have a fully coherent and self-consistent theory that explains the observations of magnetic striping, along with many other features of the Earth’s geophysics. It involves several interlocking components: convection in the metallic core producing electric currents that generate a magnetic field that is unstable over millions of years and flips polarity at irregular intervals; convection in the mantle producing upwellings of material along mid-ocean ridges, leading to seafloor spreading and continental drift; rocks that record both their age and the direction and strength of the Earth’s magnetic field when they are formed, leading to magnetic striping on the ocean beds.
Of course, this only holds together and makes sense on a spherical Earth. We’ve already seen in Proof 8. Earth’s magnetic field, that simply generating the shape of the planet’s magnetic field only works on a spherical Earth, and is an inexplicable mystery on a flat Earth model. It would be even more difficult to explain the irregular reversal of polarity of the magnetic field without a spherical core dynamo system. And plate tectonics just doesn’t work on a flat Earth either (Proof 22. Plate tectonics). Combining the fact that neither of these explanations work on a flat Earth, there is no explanation for the observed magnetic striping of the sea floors either. So magnetic striping provides another proof that the Earth is a globe.
References:
[1] Vacquier, V., Raff, A.D., Warren, R.E. “Horizontal displacements in the floor of the northeastern Pacific Ocean”. Geological Society of America Bulletin, 72(8), p.1251-1258, 1961. https://doi.org/10.1130/0016-7606(1961)72[1251:HDITFO]2.0.CO;2
[2] Mason, R.G. Raff, A.D. “Magnetic survey off the west coast of North America, 32 N. latitude to 42 N. Latitude”. Geological Society of America Bulletin, 72(8), p.1259-1265, 1961. https://doi.org/10.1130/0016-7606(1961)72[1259:MSOTWC]2.0.CO;2
[3] Raff, A.D. Mason, R.G. “Magnetic survey off the west coast of North America, 40 N. latitude to 52 N. Latitude”. Geological Society of America Bulletin, 72(8), p.1267-1270, 1961. https://doi.org/10.1130/0016-7606(1961)72[1267:MSOTWC]2.0.CO;2
[4] Heezen, B.C. “The rift in the ocean floor”. Scientific American, 203(4), p.98-114, 1960. https://www.jstor.org/stable/24940661
[5] Dietz, R.S. “Continent and ocean basin evolution by spreading of the sea floor”. Nature, 190(4779), p.854-857, 1961. https://doi.org/10.1038%2F190854a0
[6] Hess, H.H. “History of Ocean Basins: Geological Society of America Bulletin”. Petrologic Studies: A Volume to Honour AF Buddington, p.559-620, 1962. https://doi.org/10.1130/Petrologic.1962.599
[7] Vine, F.J. Matthews, D.H. “Magnetic anomalies over oceanic ridges”. Nature, 199(4897), p.947-949, 1963. https://doi.org/10.1038/199947a0
[8] Orowan, E., Ewing, M., Le Pichon, X. Langseth, M.G. “Age of the ocean floor”. Science, 154(3747), p.413-416, 1966. https://doi.org/10.1126/science.154.3747.413
[9] Müller, R.D., Sdrolias, M., Gaina, C. Roest, W.R. “Age, spreading rates, and spreading asymmetry of the world’s ocean crust”. Geochemistry, Geophysics, Geosystems, 9(4), 2008. https://doi.org/10.1029/2007GC001743
The Ancients had the technology and cleverness to work out the shape of the Earth and its diameter (see 2. Eratosthenes’ measurement). However, they had no reliable method to measure the mass of the Earth, or equivalently its density, which gives the mass once you know the volume. You could assume that the Earth has a density similar to rock throughout, but there was no way of knowing if that was correct.
In fact we had no measurement of the density or mass of the Earth until the 18th century. Perhaps surprisingly, there wasn’t even any observational evidence to decide whether the Earth was actually a solid object, or a hollow shell with a relatively thin solid crust. As late as 1692 the prominent scientist Edmond Halley proposed that the Earth might be composed of a spherical shell around 800 km thick, with two smaller shells inside it and a solid core, all separated by a “luminous” atmosphere (which could escape and cause the aurora borealis).
Structure of the Earth as proposed by Edmond Halley in 1692, with solid shells (brown) separated by a luminous atmosphere, shown in cross section.
In his 1687 publication of Philosophiæ Naturalis Principia Mathematica, Isaac Newton presented his theory of universal gravitation. Although this provided explicit equations relating the physical properties of gravitational force, mass, and size, for the cases of astronomical objects there were still more than one unknown value, so the equations could not be solved to determine the absolute masses or densities of planets. The best astronomers could do was determine ratios of densities of one planet to another.
But Newton not only proposed his formulation of gravity as a theoretical construct – he also suggested a possible experiment that could be done to test it. As observed by common experience, objects near the surface of the Earth fall downwards – they are attracted towards the centre of the Earth (more precisely, the Earth’s centre of mass). But if the attractive force of gravity is generated by mass as per Newton’s formulation, then unusual concentrations of mass should change the direction of the gravitational pull a little.
We’ve already seen in Proof 24. “Gravitational acceleration variation” that the strength of Earth’s gravitational pull varies across the Earth’s surface due to differences in altitude and density within the Earth. Now imagine a large concentration of mass on the surface of the Earth. If Newton is correct, then such a mass should pull things towards it. The attraction to the centre of the Earth is much stronger, so the direction of the overall gravitational pull should still be almost downwards, but there should be a slight deflection towards the large mass.
There are some convenient large masses on the surface of the Earth. We call them mountains. Newton conceived that one could go somewhere near a large mountain and measure the difference in angle between a plumb line (which indicates the direction of gravity, and is commonly called “vertical”) and a line pointing towards the Earth’s centre of mass (which does not have a well-defined name, since it is more difficult to measure and differs from a plumb line by an amount too small to be significant in engineering or construction – for the purposes of this proof only, I shall abbreviate it to “downwards”). However, Newton believed that any such difference would be too small to measure in practice. He writes in the Principia, Book 3: On the system of the world:
Hence a sphere of one foot in diameter, and of a like nature to the earth, would attract a small body placed near its surface with a force 20,000,000 times less than the earth would do if placed near its surface; but so small a force could produce no sensible effect. If two such spheres were distant but by 1/4 of an inch, they would not, even in spaces void of resistance, come together by the force of their mutual attraction in less than a month’s time; and lesser spheres will come together at a rate yet slower, namely in the proportion of their diameters. Nay, whole mountains will not be sufficient to produce any sensible effect. A mountain of an hemispherical figure, three miles high, and six broad, will not, by its attraction, draw the pendulum two minutes out of the true perpendicular; and it is only in the great bodies of the planets that these forces are to be perceived.[1]
Here is where Newton’s lack of experience as an experimentalist let him down. Two minutes of arc was already within the accuracies of stellar positions claimed by Tycho Brahe some 80 years earlier. If Newton had merely asked astronomers if they could measure a deflection of such a small size, they would likely have answered yes.
Engraving of Tycho Brahe observing in his observatory at Uraniborg, Sweden. (Public domain image from Wikimedia Commons.)
If you can measure how big the deflection angle is with sufficient accuracy, then you can use that measurement to calculate the density of the Earth in terms of the density of the mountain:
ρE/ρM = (VM/VE) (rE/d)2 / tan θ
where:
ρE is the density of the Earth,
ρM is the density of the mountain,
VE is the volume of the Earth,
VM is the volume of the mountain,
rE is the radius of the Earth,
d is the horizontal distance from the centre of the mountain to the plumb bob, and
θ is the angle of deflection of the plumb line from “downwards”.
The volume of the mountain can be estimated from its size and shape, and the density may be assumed to be that of common types of rock. All the other values were known, leaving the as yet unknown density of the Earth as a function of the deflection angle.
Two French astronomers, Pierre Bouguer (who we met in 4. Airy’s coal pit experiment) and Charles Marie de La Condamine, were the first to attempt to make the measurement. In 1735 they led an expedition to South America to measure the length of an arc of one degree of latitude along a line of longitude near the equator. This was part of an experiment by the French Academy of Sciences—along with simultaneous expedition to Lapland to make a similar measurement near the North Pole—to measure the shape of the Earth. Not whether it was spherical; any difference between the measurements would show if it was more accurately a prolate or an oblate ellipsoid.
Bouguer and La Condamine spent ten years on their expedition, making many other physical, geographical, biological, and ethnographical studies. One experiment they tried in 1738 was measuring the deflection of a plumb bob near the 6263 metre high volcano Chimborazo, in modern day Ecuador.
The volcano Chimborazo in Ecuador. (Creative Commons Attribution 2.0 image by David Ceballos, from Flickr.)
They climbed to an altitude of 4680 m on one flank of the mountain and 4340 m on the other side, battling harsh weather to take the two measurements. Taking two measurements on opposite sides of the mountain allows a subtraction to remove sources of error in locating the “downwards” direction, leaving behind the difference in angle between the two plumb bob directions, which is twice the desired deflection. Bouguer and La Condamine measured a deflection of 8 seconds of arc, however they considered the circumstances so difficult as to render it unreliable. But they did state that this measurement gave a large value for the Earth’s density, thus disproving the hypothesis that the Earth was hollow.
A more precise measurement of the gravitational deflection of a mountain had to wait until 1772, when Astronomer Royal Nevil Maskelyne made a proposal to repeat the experiment to the Royal Society of London.[2] The Society approved, and appointed the quaintly named Committee of Attraction to ponder the proposal. The committee (counting Joseph Banks and Benjamin Franklin among its members) despatched astronomer and surveyor Charles Mason (of Mason-Dixon line fame) to find a suitable mountain. He came back with Schiehallion, a 1083 m peak in central Scotland.
Schiehallion in central Scotland. (Creative Commons Attribution 3.0 Unported image by Wikipedia user Andrew2606, from Wikimedia Commons.)
Schiehallion had several advantages for the measurement. It’s conveniently located for a British expedition. It’s an isolated peak, with no other mountains nearby that could substantially complicate the effects of gravity in the region. It has a very symmetrical shape, making it easy to estimate the volume with some accuracy. And the northern and southern slopes are very steep, which means that by doing the experiment on those sides, the plumb bob can be positioned relatively close to the centre of mass of the mountain, increasing the deflection and making it easier to measure.
Maskelyne himself led the expedition, taking temporary leave from his post as Astronomer Royal. The party built temporary observatories on the northern and southern flanks of Schiehallion, from which they made frequent observations of overhead stars to determine the zenith line (marking the “downwards” direction), so they could compare it to the direction of the hanging plumb line. Maskelyne and his team spent 6 weeks at the southern observatory, followed by 10.5 weeks at the northern one, battling inclement weather to take the required number of observations.[3]
Map of Schiehallion and surrounds. The mountain forms a short ridge running approximately east-west. The positions of the north and south observatories can be seen. (Reproduced from [6].)
Maskelyne had calculated that if the Earth as a whole had the same density as the mountain (i.e. that of quartzite rock), then they should have observed a deflection of the plumb line relative to “downwards” of 20.9 seconds of arc. Preliminary calculations showed a deflection of about half that, meaning the Earth was roughly twice as dense as the mountain.
To mark the successful conclusion of the observations, the expedition celebrated with a rollicking good party. Plenty of alcohol was imbibed (quite possibly Scotch whisky). In the revelry, unfortunately someone accidentally set fire to the northern observatory and it burnt to the ground. The fire claimed the violin of one Duncan Robertson, a junior member of the expedition who had helped to pass the long cold nights of observation by entertaining the other members with his playing. Later, a grateful Maskelyne sent Robertson a replacement violin – not just any violin, but one made by the master craftsman Antonio Stradivari.[4][5]
The mathematician and surveyor Charles Hutton was charged with doing the detailed calculations of the result. He published them in a mammoth 100-page paper in 1778.[6] His final conclusion was that the density of the Earth was 1.8 times the density of the quartzite in Schiehallion, or about 4.5 g/cm3. Since this was so much higher than the densities of various types of rock (typically between 2 and 3 g/cm3), Hutton concluded (correctly) that much of the core of the Earth must be metal, and he calculated that about 65% of the Earth’s diameter must be a metallic core (a little higher than current measurements of 55%).
Extract of Hutton’s paper, where he states that roughly 2/3 of the diameter of the Earth must be metallic to account for the measured density. This page also shows Hutton’s calculations of the densities of solar system bodies. (Reproduced from [6].)
This was the very first time that we had any estimate of the density/mass of the Earth, and Hutton also used it to calculate the densities of the Sun, the Moon, and the planets (out to Saturn) based on their known astronomical properties, mostly to within about 20% of the modern values. So the Schiehallion experiment was groundbreaking and significantly increased our fundamental understanding of the Earth and the solar system.
Later experiments confirmed the general nature of the result and refined the figures for the density and structure of the Earth. In particular, Henry Cavendish—a chemist who 20 years earlier had discovered the elemental nature of hydrogen and made several other discoveries about air and elemental gases—turned his attention to physics and performed what has become known as the Cavendish experiment in 1797-98. He constructed a finely balanced mechanism with which he could measure the tiny gravitational attractive force between two balls of lead, which allowed the measurement of the (then unknown) value of Newton’s gravitational constant. Knowing this value, it becomes possible to directly plug in values for the size of the Earth and the acceleration due to gravity and determine the mass of the Earth. Cavendish’s result was accurate to about 1%, confirming Hutton’s conclusion that the Earth must have a core denser than rock. And then in the 20th century, seismology allowed us to confirm the existence of discrete layers within the Earth, with the central core made primarily of metal (a story for a future Proof).
Drawing of Henry Cavendish. (Creative Commons Attribution 4.0 International image by the Wellcome Collection of the British Library, from Wikimedia Commons.)
Of course, the conclusions of the Schiehallion experiment—consistent with later experiments using independent methods—depend on the fact that the Earth is very close to spherical, and the fact that gravity works as Newton said (disregarding the later refinement by Einstein, which is not significant here). One of the more popular Flat Earth models assumes that gravity does not even exist as a force, and that objects “fall” to Earth because the Flat Earth is actually accelerating upwards. In such a model, objects always fall directly “downwards” and there is no deflection caused by large masses such as mountains. The Schiehallion experiment directly and simply disproves this Flat Earth model.
If we suppose that a Flat Earth somehow manages to exist with Newtonian gravity (in itself virtually impossible, see 13. Hydrostatic equilibrium), we could posit something like the 859 km thick flat disc mentioned in 34. Earth’s internal heat. Firstly, Newtonian gravity on such a disc would not always pull perpendicular to the ground – inhabitants near the circumference would be pulled at a substantial angle towards the centre of the disc. Ignoring this, if you managed to do the Schiehallion experiment (say at the North Pole), the distance rE in the equation would be effectively 430 km (the distance to the centre of mass of the disc) rather than the radius of the spherical Earth, 6378 km. This should make the observed deflection angle approximately (6378/430)2 = 220 times smaller! Then the observed deflections would imply that the density of the Earth is 220 times higher, or around 990 g/cm3, about 6 times as dense as the core of the Sun. Which is then inconsistent with the assumed density being the same as the spherical Earth (among other problems).
On the other hand, if we allow the density to be a free parameter, we can solve the gravitational and geometric equations simultaneously to derive the thickness of the Flat Earth disc in a “consistent” manner. This produces a thickness of 3020 km, and a density of 92 g/cm3. Which is over 4 times as dense as osmium, the densest substance at non-stellar pressures. So we’ve shown that the Schiehallion experiment proves that this “Newtonian Flat Earth” model cannot possibly be composed of any known material.
Basically, the observations of the Schiehallion experiment cannot be made consistent with a flat Earth, thus providing evidence that the Earth is a globe.
References:
[1] Newton, I. Philosophiae Naturalis Principia Mathematica (1687). Trans. Andrew Motte, 1729.
[2] Maskelyne, N. “A proposal for measuring the attraction of some hill in this Kingdom”. Philosophical Transactions of the Royal Society, 65, p. 495-499, 1772. https://doi.org/10.1098/rstl.1775.0049
[3]. Sillitto, R.M. “Maskelyne on Schiehallion: A Lecture to The Royal Philosophical Society of Glasgow”. 1990. http://www.sillittopages.co.uk/schie/schie90.html
[4] Davies, R. D. “A Commemoration of Maskelyne at Schiehallion”. Quarterly Journal of the Royal Astronomical Society, 26, p. 289, 1985. https://ui.adsabs.harvard.edu/abs/1985QJRAS..26..289D
[5] Danson, E. Weighing the World. Oxford University Press, 2005. ISBN 978-0-19-518169-2.
[6] Hutton, C. “An Account of the Calculations made from the Survey and Measures taken at Schehallien, in order to ascertain the mean Density of the Earth”. Philosophical Transactions of the Royal Society. 68, p. 689-788, 1778. https://doi.org/10.1098/rstl.1778.0034
I haven’t posted a new proof in a while, and that will probably continue for a few more weeks. I currently have a bandaged up left hand due to a kitchen knife accident, which is making it slower for me to work on the computer. And I also have my wife working from home due to COVID-19 travel restrictions, which breaks up my days with many more distractions than normal, making it very hard to concentrate in the long blocks of time necessary to research and write up a new proof.
Rest assured that the site is not abandoned! I’ll be back with more new proofs, hopefully within a few weeks.
A waveguide is a structure that restricts the motion of waves, disallowing propagation in certain directions, and thus concentrating the energy of the wave to propagate in specific other directions. An example of a waveguide is an optical fibre, which is basically a long, thin string of flexible glass or transparent polymer. Light entering one end is channelled along the fibre, unable to escape from the sides, and emerges at almost the same brightness from the far end.
Normally light and other electromagnetic waves, as well as other waves such as sound, spread out in three dimensions. As the energy spreads out to cover more space, conservation of energy causes the power to fall off according to the inverse square law: power falls as the reciprocal of the square of the distance from the source.
With a waveguide, propagation of the wave can be restricted to a single dimension so the energy doesn’t spread out, resulting in all of the energy being transmitted to the far end (minus a small fraction that may be absorbed or otherwise lost along the way). Sound waves, for example, can be guided by simple hollow tubes, the sound preferring to propagate along the interior air channel than penetrate the tube walls. This is the principle behind medical stethoscopes and old fashioned speaking tube systems.
Another type of waveguide is a transmission line, which is a pair of electrical cables used to transmit alternating current (AC) electrical power. The cables can simply be parallel wires in close proximity, or a coaxial cable, in which an insulated wire runs down the core of tubular conductor. Domestic AC power has a frequency of 50 to 60 hertz, which is low compared to the kilohertz range of radio frequencies. Transmission lines can carry electromagnetic waves up to frequencies of around 30 kHz. Above this, paired wires start to radiate radio waves, so they become inefficient and a different type of waveguide is used.
Radio waveguides are commonly hollow metal tubes. Radio waves travel along the tube, and the conductive metal prevents the waves from leaking to the outside. Such waveguides are used to transmit radio power in radar systems and microwaves in microwave ovens. Anywhere there is a cavity bounded by regions that waves cannot pass through, a waveguide effect can be generated.
Radio waves travel easily through the Earth’s atmosphere, to and from transmission towers and the various wireless devices we use. However the bulk of the Earth is opaque to radio waves; you generally need a mostly unobstructed line of sight, barring relatively thin obstructions like walls.
But there is another region of the Earth that is opaque to (at least some) radio waves. The ionosphere is the region of the atmosphere in which incoming solar radiation ionises the atmospheric gases (mentioned previously in 31. Earth’s atmosphere). It lies between approximately 60 to 1000 km altitude. Since ionised gas conducts electricity, low frequency radio waves cannot pass through it (higher frequencies oscillate too rapidly for the ionised particles to respond).
Radio waves with wavelengths longer than about 30 metres—or frequencies below about 10 MHz—are thus trapped in the atmosphere between the Earth’s surface and the ionosphere. This forms a waveguide which can carry so-called shortwave radio signals around the world, alternately bouncing off the ionosphere and the Earth’s surface.
There are also natural sources of low frequency radio waves. Lightning flashes in storm systems produce huge discharges of electrical energy, and the sudden release of this energy generates radio waves. If you’ve ever listened to a radio during a thunderstorm you’ll be familiar with the bursts of static caused by strokes of lightning. Lightning generates broadband radio emissions, meaning it covers a wide range of radio frequencies, including the very low frequencies that are guided by the ionospheric waveguide.
Atmospheric scientists measure the amount of lightning around the world by monitoring tiny changes in the Earth’s magnetic field, of the order of picoteslas, caused as these radio waves pass by. The sensitive detectors they use can detect lighting strikes anywhere on the planet. There are a few specific radio frequencies at which the lightning strikes turn out to be especially strong. The following plot shows the intensity of magnetic field fluctuations as a function of radio frequency.
The first peak in the observed radio spectrum is at 7.8 Hz, followed by peaks at 14.3 Hz, 20.8 Hz, and roughly every 6.5 Hz thereafter. People familiar with wave theory will recognise from the pattern that these are likely resonance frequencies, with a fundamental mode at 7.8 Hz, followed by overtones. A wave resonance occurs when an exact number of wavelengths fits into a confined cavity. The wave propagates and bounces around and, because of the precise match with the cavity size, reflected waves end up with peaks and troughs in the same physical position, reinforcing one another. So at the specific resonance frequency, the wave builds up in intensity, while at other frequencies the waves self-interfere and rapidly die down. These resonance frequencies, which are measured at many research stations around the world, are known as Schumann resonances.
The Irish physicist George Francis FitzGerald first anticipated the existence of Schumann resonances in 1893, but his work was not widely circulated. Around 1950, the German physicist Winfried Otto Schumann performed the theoretical calculations that predicted the resonances may be observable, and made efforts to observe them. But it was not until 1960 that Balser and Wagner made the first successful observations and measurements of Schumann resonances.[2]
What causes the radio waves produced by lightning flashes to have a resonance at 7.8 Hz? Well, radio waves travel at the speed of light, so let’s divide the speed of light by 7.8 to see what the wavelength is: the answer is 38,460 km. If you’ve been paying attention to many of these articles, you’ll realise that this is very close to the circumference of the Earth.
Radio waves with a frequency of 7.8 Hz are travelling around the world in the waveguide formed by the Earth and the ionosphere, and returning one wavelength later to constructively interfere and reinforce themselves, producing a measurable peak in Earth’s magnetic field fluctuations at 7.8 Hz. The resonance peak is broad and a little different to 7.5 Hz (the speed of light divided by the circumference of the Earth) because the geometry of a spherical cavity is more complicated than a simple circular loop – effectively some propagation paths are shorter because the waves don’t all take a great circle route.
So Schumann resonances are an observed phenomenon that has a natural explanation – if the Earth is a globe.
If the Earth were flat, then any ionosphere above it would be flat as well, and would still form a waveguide for low frequency radio waves. However it would not be a closed waveguide. Radio waves would propagate out the edges and be lost to space, meaning there would be no observable magnetic field resonances at all. And even if there were an opaque radio wall of some sort at the edge of the flat Earth, the size and geometry of the resulting cavity would be different, resulting in a different set of resonance frequencies, more akin to the frequencies of a vibrating disc, which are not evenly spaced like the observed Schumann resonances.
And so Schumann resonances provide another proof that the Earth is a globe.
References:
[1] Shanmugam, M. “Investigation of Near Earth Space Environment”. Ph.D. Thesis, Manonmaniam Sundaranar University, 2016. https://www.researchgate.net/publication/309209580_Investigation_of_Near_Earth_Space_Environment
[2] Balser, M., Wagner, C. “Observations of Earth–Ionosphere Cavity Resonances”. Nature, 188, p. 638-641, 1960. https://doi.org/10.1038/188638a0
The French physicist Henri Becquerel discovered the phenomenon of radioactivity in 1896, while performing experiments on phosphorescence – the unrelated phenomenon that causes “glow in the dark” materials to glow for several minutes after being exposed to light. He was interested to see if phosphorescence was related to x-rays, discovered only a few months earlier by Wilhelm Roentgen. In his experiments, Becquerel noticed that uranium salts could darken photographic film, even if wrapped in black paper so that no light could fall on the film, and even from non-phosphorescent uranium samples. The conclusion was that some sort of penetrating rays were being emitted by the uranium itself, without being excited by external energy.
Marie and Pierre Curie quickly discovered other radioactive elements, and Becquerel himself discovered by experimenting with magnets that there were three different types of radioactive radiation: two deflected in different directions by a magnetic field and one not deflected at all. In 1899, Ernest Rutherford characterised the first two types, naming them alpha and beta particles, with positive and negative electric charges. Becquerel measured the mass/charge ratio of beta particles in 1900 and determined that they were the same as the electrons discovered by J. J. Thomson in 1897. In 1907 Rutherford showed that alpha particles were the nuclei of helium atoms. And in 1914, he showed that the third type of radiation, named gamma rays, were a form of electromagnetic radiation.
This was an exciting time in physics, and our understanding of atomic structure was revolutionised within the space of two decades. Besides discovering the basic structure of the atom and how it related to the phenomenon of radioactive decay, several peripheral phenomena also came to the attention of scientists.
One observation was that atoms in the atmosphere were sometimes ionised, or “electrified” as the scientists of the time described it. Ionisation is the process of electrons being stripped off neutral atoms, to form negatively charged free electrons and positively charged atomic ions (consisting of the atomic nucleus and a less-than-full complement of electrons). It was clear that radioactive rays could ionise atoms in the air, and so scientists assumed that it was radiation from radioactive elements in the ground that was ionising the air near ground level.
Except strangely the amount of ionising radiation in the atmosphere seemed to increase with increasing altitude. German physicist and Jesuit priest Theodor Wulf invented in 1909 a portable electroscope capable of measuring the ionisation of the atmosphere. He used it to investigate the source of the ionising radiation by measuring ionisation at the base and the top of the Eiffel Tower. He found that the ionisation at the top of the 300 metre tower was a bit over half that at ground level, which was higher than he expected, since theoretically he expected the ionisation to drop by half every 80 metres, so to be less than one tenth the ionisation at ground level. He concluded that there must be some other source of ionising radiation coming from above the atmosphere. However, his published paper was largely ignored.
In 1911, the Italian physicist Domenico Pacini measured the ionisation rates in various places, including mountains, lakes, seas, and underwater. He showed that the rate dropped significantly underwater, and concluded that the main source of radiation could not be the Earth itself. Then in 1912, Austrian physicist Victor Hess took some Wulf electroscopes up in a hot air balloon to altitudes as high as 5300 metres, flying both in daylight, night time, and during an almost complete solar eclipse.
Hess showed that the amount of ionising radiation decreased as one moved from ground level up to about 1000 metres, but then increased again rapidly. At 5300 metres, there was approximately twice as much ionising radiation as at ground level.[1] And because the effect occurred at night, and during a solar eclipse, it wasn’t due to the sun. Hess had proven that there was a source of this radiation outside the Earth’s atmosphere. Further unmanned balloon flights as high as 9 km showed the radiation increased even higher with altitude.
What this mysterious radiation was remained unknown until the late 1920s. It was initially thought to be electromagnetic radiation (i.e. gamma rays and x-rays). Robert Millikan named them cosmic rays in 1925 after proving that they originated outside the Earth. Then in 1927 the Dutch physicist Jacob Clay performed measurements while sailing from Java to the Netherlands, which showed that their intensity increased as one moved from the tropics to mid-latitudes.[2] He correctly deduced that the intensity was affected by the Earth’s magnetic field, which implied the cosmic rays must be charged particles.
In 1930, the Italian Bruno Rossi realised that if cosmic rays are electrically charged, then they should be deflected either east or west by the Earth’s magnetic field, depending on whether they are positively or negatively charged, respectively.[3] Experiments found that at all locations on the Earth’s surface there are more cosmic rays coming from the west than from the east, showing that most (if not all) cosmic ray particles are positively charged. This observation was called the east-west effect.
Subsequent experiments determined that around 90% of cosmic rays entering our atmosphere are protons, 9% are helium nuclei (or alpha particles), and the remaining 1% are nuclei of heavier elements, with an extremely small number of other types of particles. And in 1936, for his crucial part in the discovery in cosmic rays, Victor Hess was awarded the Nobel Prize for Physics.
The origin of cosmic rays is however still not entirely clear. Our sun produces energetic particles that reach Earth, but cosmic rays are generally defined as coming from outside our own solar system. Our Milky Way Galaxy produces some of the lower energy particles, mostly from the direction of the galactic core, however at very high energies there is a deficit of cosmic rays in that direction, implying a shadowing effect on rays whose origin lies outside our galaxy. Known sources of cosmic rays include supernova explosions, supernova remnants (such as the Crab Nebula), active galactic nuclei, and quasars. But there are some very high energy cosmic rays whose source is still a mystery.
The fact that high energy cosmic rays originate from outside our galaxy, means that they should be isotropic – uniform in intensity distribution, independent of the direction from which they approach Earth. However, the Earth is not a stationary observation platform. The Earth orbits the sun at a speed of almost 30 km/s. But on a galactic scale this motion is dwarfed by the sun’s orbital speed around the core of our galaxy, which is 230 km/s, roughly in the direction of the star Vega, in the constellation Lyra. So relative to extragalactic cosmic rays, Earth is moving at an average speed of approximately 230 km/s. This speed adds to the energy of cosmic rays coming from the direction of Vega, and subtracts from the energies of cosmic rays coming from the opposite direction.
This difference is known as the Compton-Getting effect after the discoverers Arthur Compton and Ivan Getting.[4] It produces about a 0.1% difference in the energies of cosmic rays coming from the opposite directions, which can be observed statistically. The effect was confirmed experimentally in 1986.[5]
So we have two different observational effects that have been experimentally confirmed in the distribution of cosmic rays arriving at Earth. The Compton-Getting effect shows that the Earth is moving in the direction of the star Vega. Vega is of course above the Earth’s horizon as seen from half the planet’s surface at any one time, and below the horizon (behind the planet) from the other half of the Earth’s surface. By measuring cosmic ray distributions, you can show that the direction defined by the Compton-Getting anisotropy relative to the ground plane varies depending on your position on Earth. In other words, by measuring cosmic rays, you can prove that the Earth’s direction of motion through the galaxy is upwards from the ground in one place, while simultaneously downwards into the ground from a point on the opposite side of the planet, and at intermediate angles in places in between. Which is perfectly consistent for a spherical planet, but inconsistent with a Flat Earth.
The second effect, the east-west effect, is also readily explained with a spherical Earth, with the addition of a simple dipole magnetic field. As can be seen in the diagram above (“Illustration of the east-west effect”), incoming positively charged cosmic rays are uniformly deflected to the right (as viewed from above Earth’s North Pole), resulting in more rays arriving from the west than from the east, independent of location or time of day. The same observed east-west effect could in theory be produced on a Flat Earth, but only if the magnetic field is flattened out as well, holding the same relative orientation to the Earth’s surface as it does on the globe.
This would result in the field being grossly distorted from that of a simple dipole, and thus requiring some exotic method of generating such a complex field – a complex field that just happens to mimic exactly the field of a straightforward dipole if the Earth were spherical. In another application of Occam’s razor (similar to its use in article 8. Earth’s magnetic field), it is more parsimonious to conclude that the Earth is not flat, but spherical.
References:
[1] Hess, V.F. “Über Beobachtungen der durchdringenden Strahlung bei sieben Freiballonfahrten” (Observation of penetrating radiation in seven free balloon flights). Physikalische Zeitschrift, 13, p.1084-1091, 1912. http://inspirehep.net/record/1623161/
[2] Clay, J. “Penetrating radiation”. Proceedings of the Royal Academy of Sciences Amsterdam, 30, p. 1115-1127, 1927. https://www.dwc.knaw.nl/DL/publications/PU00011919.pdf
[3] Rossi, B. “On the magnetic deflection of cosmic rays”. Physical Review, 36(3), p. 606, 1930. https://doi.org/10.1103/PhysRev.36.606
[4] Compton, A. H., Getting, I. A. “An Apparent Effect of Galactic Rotation on the Intensity of Cosmic Rays”. Physical Review, 47, p. 817-821, 1935. https://doi.org/10.1103/PhysRev.47.817
[5] Cutler, D., Groom, D. “Observation of terrestrial orbital motion using the cosmic-ray Compton–Getting effect”. Nature, 322, p. 434-436, 1986. https://doi.org/10.1038/322434a0
